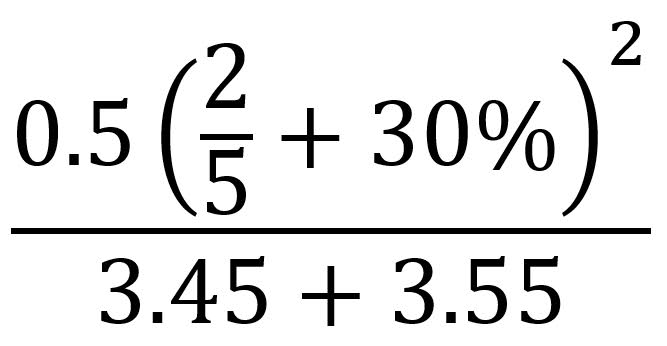GUEST POST: Building Effective Learning Strategies into a Mathematics Curriculum
(cover image by michael davis-burchat; NoDerivs 2.0 Generic (CC BY-ND 2.0)
By Jemma Sherwood
Jemma Sherwood is Head of Mathematics in a Secondary School in England. She blogs here and is on Twitter as @jemmaths.
Last year I started planning a scheme of work for our mathematics department with a couple of aims in mind. Firstly, I wanted it to follow the principles of a mastery curriculum (as explained here) and, secondly, I wanted to embed the learning principles I had become familiar with through the Learning Scientists. Our curriculum covers the five years of compulsory mathematics in Secondary school for 11-16 year olds, culminating in the GCSE exam, an exam which has been made harder in recent years with an increase in content and the requirement to learn key formulae by heart (readers outside England, see here for more information on our education system).
We began the curriculum in September with Years 7, 8 and 9 students and it is very much a work in progress. Every element, from the sequencing of topics through to the feedback we give to students, is being considered alongside research and evidence wherever possible and we are using this research to inform discussion in our department development meetings. We don’t have a finished product yet (could anyone ever say that in education?), but I believe we are on the right journey.
Here is how we have started to build in the six strategies for effective learning.
Spaced Practice and Interleaving
I considered these two strategies together when planning the scheme of work, as the implementation of both follows broadly the same principles. How we do this is best illustrated with an example.
In Units N2 and N3 (Addition, Subtraction, Multiplication and Division) students learn how to perform these operations on positive integers and decimals. Later on, in unit N5, they learn about the order of operations (brackets/parentheses before indices, etc.) and while practicing this they complete both the standard “traditional” questions involving integers, but also questions involving decimals as a way of reinforcing their earlier learning. The principle of returning to previously studied content and revisiting it in a new context continues from here. In Unit N6 (Negative Numbers) students use negative integers and decimals in order of operations questions and in Unit N7 (Fractions) – once students have learned how to add, subtract, multiply, and divide fractions – they are challenged with order of operations questions again.
Once Unit N8 (Percentages) is underway, students have practiced converting between fractions, decimals, and percentages and can then tackle convoluted questions such as:
When we begin to study algebra, we come to topics such as substitution and solving equations and, yet again, students will have to practice working with decimals, fractions, negative numbers and order of operations.
It is very easy to teach many of these topics in isolation, sticking to working with integers in the majority of cases. But by giving students regular, spaced exposure to material they have learned previously, and in increasingly complex situations, we allow them the opportunity to remember what they may have forgotten (retrieval practice) and embed their learning more deeply.
Another way of interleaving content is something that happens in math almost automatically due to its hierarchical nature. One topic can become increasingly complex and is not always taught in its entirety at a single time. Our Unit A4 (The Cartesian Grid) features plotting linear graphs and comparing their gradient and y-intercept to the equation of the graph. A year later students will study A7 (Advanced Linear Graphs and Equations) where they will work with perpendicular and parallel lines as well as solving simultaneous equations graphically. This naturally builds on their previous learning. Our aim is that, in spacing these two units and distributing practice between them, students have not forgotten so much that they need to be completely retaught.
Retrieval Practice
As well as interleaving the practice of topics, we use regular low-stakes quizzing on previously learned content to practice retrieval. Students are given a knowledge organizer at the start of each unit, which sets out the minimum we expect them to learn by the end of the unit. This may include key mathematical facts, such as the prefixes on the SI units or some of the most common fraction-decimal-percentages conversions like eighths, thirds and fifths. These are the kinds of topics for which we want to achieve quick recall, just like we would with the times tables, and regular quizzing helps students to remember these facts.
Combine this with homework tasks throughout the year that review earlier learning, and students are given plenty of opportunity to retrieve what they have learned. This year we have used the excellent Numeracy Ninjas resources to good effect with students aged 11-14, and we are now investigating something similar we could use with the older students.
Elaboration
As teachers we strive to make links between topics and help students to see the bigger picture that is so obvious to us but not to them as novices. Elaboration in mathematics is essential to better learning, because it is important that students don’t just learn a set of facts or rules but that they acquire a deeper understanding of how the ideas they encounter fit together. For instance, algebraic techniques link to numerical ones: factorising (or ‘factoring’ in the US) an algebraic expression can be compared to factorising numbers, simplifying an algebraic fraction can be compared to simplifying a numerical fraction.
Getting the balance between simplicity, or clarity, of instruction and elaboration is an important one and this balance varies between topics and between classes. Looking for ways to improve instruction allows for more opportunities for elaboration, which is where the last two strategies come in.
Concrete Examples
Concrete examples are the bedrock of mathematics instruction. Trying to explain a concept without giving worked examples can be like talking in a foreign language, and every math teacher knows that examples are essential. The important thing is the way you choose your examples. Do your examples help teach the main idea, or do they detract from it?
For instance, when teaching students to solve equations I want to present them with equations that they could not solve just by sight, as they will not see the need for learning the procedure. When I am teaching them to rearrange a formula I need to break down all the different types of procedure involved and present these carefully and sequentially, giving the students practice time between examples. I must always remember that I am inclined to suffer from the curse of knowledge: it is easy to overestimate what my students can do when I find it so easy.
Dual Coding
Certain areas of mathematics lend themselves to dual coding. Solving geometric problems is the obvious one, but we don’t always take advantages of the benefits of dual coding: it is more than simply showing an image to accompany a problem. Consider the following example, taken from the Edexcel Mathematics GCSE Paper 1MA0/1H from June 2012, presented in three different ways:
Version 1: The question presented only in words
Version 2: The question presented in words with an accompanying diagram (as it was in the examination)
Version 3: The question presented as an annotated diagram
When first learning circle theorems students may find it easier to process the scenario when presented as version three. As a teacher I would show the diagram and introduce each statement one after the other, with arrows, and then remove the arrows (which are a little distracting) once the problem is set up. While teaching students how to solve such a problem I would annotate the diagram as we go along, finding as many missing angles as possible and helping the students to decide which angles they do and don’t need to know in order to solve the problem.
As students become more expert, versions 2, then 1, become more accessible and I must make a judgment about when to use each type of presentation and how to guide students to the point where they can construct their own diagrams from words alone. This dual coding principle can be used across a huge variety of mathematical topics.
How do we know if all this is working?
As this curriculum is different from what we have done previously, from lessons through to assessments, we have very few ways of making objective judgments about its success, comparing these cohorts to previous ones. What I can say, anecdotally, is that I am finding my classes’ ability to remember previously learned content much better than in the past and feedback from the other teachers in the department is positive. Ultimately, time will tell as more of the students on this pathway go through the GCSE exams. For now I am confident that we are moving in the right direction, thinking explicitly about how to improve our students’ knowledge and memory in addition to merely teaching the math.